Complement of Binary Numbers
Complement of Binary Numbers
Complements are used in computer for the simplification of the subtraction operation. For any number in base r, there exist two complements—(1) r’s complement and (2) r-1 ’s complement.
| Number System | Base | Complements Possible |
|---|---|---|
| Binary | 2 | 1’s complement and 2’s complement |
| Octal | 8 | 7’s complement and 8’s complement |
| Decimal | 10 | 9’s complement and 10’s complement |
| Hexadecimal | 16 | 15’s complement and 16’s complement |
Signed and Unsigned Numbers
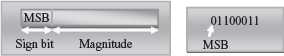
A binary number may be positive or negative. Generally, we use the symbol “+” and “−” to represent positive and negative numbers, respectively. The sign of a binary number has to be represented using 0 and 1, in the computer. An n-bit signed binary number consists of two parts—sign bit and magnitude. The left most bit, also called the Most Significant Bit (MSB) is the sign bit. The remaining n-1 bits denote the magnitude of the number.
In signed binary numbers, the sign bit is 0 for a positive number and 1 for a negative number.
For example, 01100011 is a positive number since its sign bit is 0, and, 11001011 is a negative number since its sign bit is 1. An 8–bit signed number can represent data in the range −128 to +127 (–27to +27−1). The left-most bit is the sign bit.
In an n-bit unsigned binary number, the magnitude of the number n is stored in n bits. An 8–bit unsigned number can represent data in the range 0 to 255 (28 = 256).
1’s Complement of Binary Number
1’s Complement of Binary Number is computed by changing the bits 1 to 0 and the bits 0 to 1
Example
» 1’s complement of 101 is 010
» 1’s complement of 1011 is 0100
» 1’s complement of 1101100 is 0010011
2’s Complement of Binary Number
2’s Complement of Binary Number is computed by adding 1 to the 1’s complement of the binary number.
Example
» 2’s complement of 101 is 010 + 1 = 011
» 2’s complement of 1011 is 0100 + 1 = 0101
» 2’s complement of 1101100 is 0010011 + 1 = 0010100
The rule to find the complement of any number N in base r having n digits is
(r − 1)’s complement — (rn − 1) − N
(r’s) complement —(rn − 1) − N + 1 = (rn − N)
